341 Triangles with 33 Lines

In 2008, Jérémie Blanc informed me about the following paper, which demonstrates that for infinitely many numbers k, perfect Kobon triangle solutions exist (configurations yielding the maximum number of triangles).

authors

D. Forge and J. L. Ramirez Alfonsin

title

Straight line arrangements in the real projective plane

publication

Discrete and Computational Geometry, volume 20, number 2, pages 155-161, 1998

url

Online Access

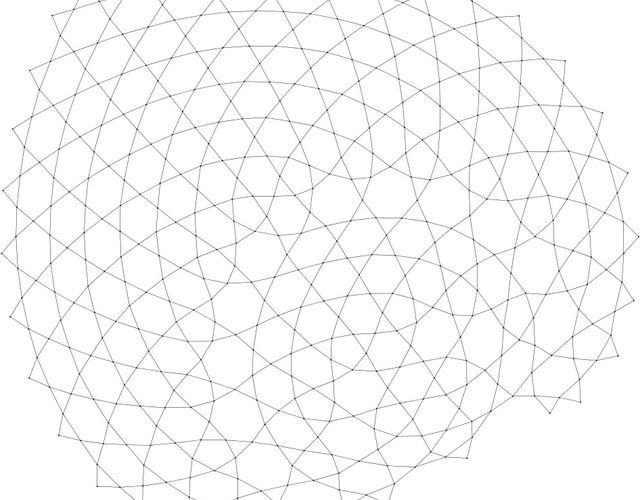
I implemented the method of Forge and Alfonsin to create the optimal arrangement of 33 lines (yielding 341 triangles), in three steps given the maximal configuration of 5 lines. You can download the code here.

The following image shows the line intersection graph that represents the arrangement of 33 lines. Every circle of three vertices represents one of the 341 triangles in the line arrangement.